module 4 lesson 25 homework 5th grade

Texas Go Math
Big Ideas Math
Engageny Math
McGraw Hill My Math
enVision Math
180 Days of Math
Math in Focus Answer Key
Math Expressions Answer Key
Privacy Policy

$CCSS Math Answers$

Eureka Math Grade 5 Module 4 Lesson 25 Answer Key

Engage ny eureka math 5th grade module 4 lesson 25 answer key, eureka math grade 5 module 4 lesson 25 problem set answer key.

$Eureka Math Grade 5 Module 4 Lesson 25 Problem Set Answer Key 1$

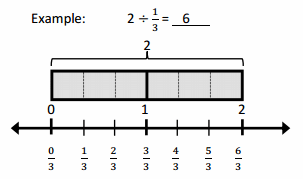
a. 4 ÷ $\frac{1}{2}$ = _________ There are __ halves in 1 whole. There are ___ halves in 4 wholes. If 4 is $\frac{1}{2}$, what is the whole? __

Answer: 4 ÷ $\frac{1}{2}$ = 8 There are 2 halves in 1 whole. There are 8 halves in 4 wholes. If 4 is $\frac{1}{2}$, the whole is 4.

$Eureka-Math-Grade-5-Module-4-Lesson-25-Problem-Set-Answer-Key-1-2$

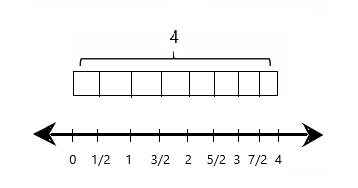
b. 2 ÷ $\frac{1}{4}$ = __ There are ____ fourths in 1 whole. There are ____ fourths in 2 wholes. If 2 is $\frac{1}{4}$, what is the whole? __

Answer: 2 ÷ $\frac{1}{4}$ = 8 There are 4 fourths in 1 whole. There are 8 fourths in 2 wholes. If 2 is $\frac{1}{4}$, the whole is 8

Explanation: Given that 2 ÷ $\frac{1}{4}$ on solving we will get the result as 8. And there are 4 fourths in 1 whole and there are 8 fourths in 2 wholes.

c. 5 $\frac{1}{3}$ = __ There are ____ thirds in 1 whole. There are ____ thirds in 5 wholes. If 5 is $\frac{1}{3}$, what is the whole? __

Answer: 5 $\frac{1}{3}$ = 15 There are 3 thirds in 1 whole. There are 15 thirds in 5 wholes. If 5 is $\frac{1}{3}$, the whole is 15.

Explanation: Given that 5 $\frac{1}{3}$ on solving we will get the result as 15. And there are 3 thirds in 1 whole and there are 15 thirds in 5 wholes.

d. 3 ÷ $\frac{1}{5}$ = _________ There are ____ fifths in 1 whole. There are ____ fifths in 3 wholes. If 3 is $\frac{1}{5}$, what is the whole? __

Answer: 3 ÷ $\frac{1}{5}$ = 15 There are 5 fifths in 1 whole. There are 15 fifths in 3 wholes. If 3 is $\frac{1}{5}$, the whole 15.

Explanation: Given that 3 ÷ $\frac{1}{5}$ on solving we will get the result as 15. And there are 5 fifths in 1 whole and tThere are 15 fifths in 3 wholes.

Question 3. Divide. Then, multiply to check.

a. 5 ÷ $\frac{1}{2}$

Answer: 5 ÷ $\frac{1}{2}$ = 10.

Explanation: Given that 5 ÷ $\frac{1}{2}$ which is 5 × 2 = 10. To check we will $\frac{1}{2}$ × 10 which is 5.

b. 3 ÷ $\frac{1}{2}$

Answer: 3 ÷ $\frac{1}{2}$ = 6.

Explanation: Given that 3 ÷ $\frac{1}{2}$ which is 3 × 2 = 6. To check we will $\frac{1}{2}$ × 6 which is 3.

c. 4 ÷ $\frac{1}{5}$

Answer: 4 ÷ $\frac{1}{5}$ = 20.

Explanation: Given that 4 ÷ $\frac{1}{5}$ which is 4 × 5 = 20. To check we will $\frac{1}{5}$ × 20 which is 4.

d. 1 ÷ $\frac{1}{6}$

Answer: 1 ÷ $\frac{1}{6}$ = 6.

Explanation: Given that 1 ÷ $\frac{1}{6}$ which is 1 × 6 = 6. To check we will $\frac{1}{6}$ × 6 which is 1.

e. 2 ÷ $\frac{1}{8}$

Answer: 2 ÷ $\frac{1}{8}$ = 16.

Explanation: Given that 2 ÷ $\frac{1}{8}$ which is 2 × 8 = 16. To check we will $\frac{1}{8}$ × 16 which is 2.

f. 7 ÷ $\frac{1}{6}$

Answer: 7 ÷ $\frac{1}{6}$ = 42.

Explanation: Given that 7 ÷ $\frac{1}{6}$ which is 7 × 6 = 42. To check we will $\frac{1}{6}$ × 42 which is 7.

g. 8 ÷ $\frac{1}{3}$

Answer: 8 ÷ $\frac{1}{3}$ = 24.

Explanation: Given that 8 ÷ $\frac{1}{3}$ which is 8 × 3 = 24. To check we will $\frac{1}{3}$ × 24 which is 8.

h. 9 ÷ $\frac{1}{4}$

Answer: 9 ÷ $\frac{1}{4}$ = 36.

Explanation: Given that 9 ÷ $\frac{1}{4}$ which is 9 × 4 = 36. To check we will $\frac{1}{4}$ × 36 = 9.

Question 3. For an art project, Mrs. Williams is dividing construction paper into fourths. How many fourths can she make from 5 pieces of construction paper?

Answer: The number of fourths can she make from 5 pieces of construction paper is 20 fourths.

Explanation: Here, Mrs. Williams is dividing construction paper into fourths, so the number of fourths can she make from 5 pieces of construction paper is 5 ÷ $\frac{1}{4}$ which is 5 × 4 = 20.

Question 4. Use the chart below to answer the following questions. Donnie’s Diner Lunch Menu

a. How many hamburgers can Donnie make with 6 pounds of hamburger meat?

Answer: The number of hamburgers can Donnie make with 6 pounds of hamburger meat is 18 hamburgers.

Explanation: The number of hamburgers can Donnie make with 6 pounds of hamburger meat is 6 ÷ $\frac{1}{3}$ which is 6 × 3 = 18 hamburgers.

b. How many pickle servings can be made from a jar of 15 pickles?

Answer: The number of pickle servings can be made from a jar of 15 pickles is 60 pickles.

Explanation: The number of pickle servings can be made from a jar of 15 pickles is 15 ÷ $\frac{1}{4}$ which is 15 × 4 = 60 pickles

c. How many servings of chocolate milk can he serve from a gallon of milk?

Answer: The number of servings of chocolate milk can he serve from a gallon of milk is 32 servings of chocolate milk..

Explanation: The number of servings of chocolate milk can he serve from a gallon of milk is, as 1 gallon is 16 cups and 16 ÷ $\frac{1}{2}$ which is 16 × 2 = 32 servings of chocolate milk.

Question 5. Three gallons of water fill $\frac{1}{4}$ of the elephant’s pail at the zoo. How much water does the pail hold?

Answer: The pail holds 12 gallons.

Explanation: Here, Three gallons of water fill $\frac{1}{4}$ of the elephant’s pail at the zoo, so the pail holds 3 ÷ $\frac{1}{4}$ which is 3 × 4 = 12 gallons.

Eureka Math Grade 5 Module 4 Lesson 25 Exit Ticket Answer Key

Question 1. Draw a tape diagram and a number line to solve. Fill in the blanks that follow.

a. 5 ÷ $\frac{1}{2}$ = _________ There are ____ halves in 1 whole. There are ____ halves in 5 wholes. 5 is $\frac{1}{2}$ of what number? _______

Answer: 5 ÷ $\frac{1}{2}$ = 10 There are 2 halves in 1 whole. There are 10 halves in 5 wholes. 5 is $\frac{1}{2}$ the number is 10

Explanation: Given that 5 ÷ $\frac{1}{2}$ which is 5 × 2 = 10. And there are 2 halves in 1 whole and there are 10 halves in 5 wholes.

b. 4 ÷ $\frac{1}{4}$ = _________ There are ____ fourths in 1 whole. There are ____ fourths in ____ wholes. 4 is $\frac{1}{4}$ of what number? _______

Answer: 4 ÷ $\frac{1}{4}$ = 16 There are 4 fourths in 1 whole. There are 16 fourths in 4 wholes. 4 is $\frac{1}{4}$ the number 16

Explanation: Given that 4 ÷ $\frac{1}{4}$ which is 4 × 4 = 16. And there are 4 fourths in 1 whole and there are 16 fourths in 4 wholes.

Question 2. Ms. Leverenz is doing an art project with her class. She has a 3 foot piece of ribbon. If she gives each student an eighth of a foot of ribbon, will she have enough for her class of 22 students?

Answer: Ms. Leverenz has 3 foot ribbon or 36 inches, so she have enough for her class 22 students.

Explanation: Here, Ms. Leverenz is doing an art project with her class and she has a 3 foot piece of ribbon, which is 12 × 3 = 36 foot. and if she gives each student an eighth of a foot of ribbon which is $\frac{8}{12}$ = 1.5 inches of ribbon. Here Ms. Leverenz gives each students 1.5 inches ribbon. Therefore she needs for 22 students which is 22 × 1.5 = 33 inches. So she has 3 foot ribbon or 36 inches, so she have enough for her class 22 students.

Eureka Math Grade 5 Module 4 Lesson 25 Homework Answer Key

Question 1. Draw a tape diagram and a number line to solve. Fill in the blanks that follow. a. 3 ÷ $\frac{1}{3}$ = _________ There are ____ thirds in 1 whole. There are ____ thirds in 3 wholes. If 3 is $\frac{1}{3}$, what is the whole? _______

Answer: 3 ÷ $\frac{1}{3}$ = 9 There are 3 thirds in 1 whole. There are 9 thirds in 3 wholes. If 3 is $\frac{1}{3}$, the whole is 9.

Explanation: Given that 3 ÷ $\frac{1}{3}$ which is 3 × 3 = 9. And there are 3 thirds in 1 whole and there are 9 thirds in 3 wholes.

b. 3 ÷ $\frac{1}{4}$ = _________ There are ____ fourths in 1 whole. There are ____ fourths in __ wholes. If 3 is $\frac{1}{4}$, what is the whole? _______

Answer: 3 ÷ $\frac{1}{4}$ = 12 There are 4 fourths in 1 whole. There are 12 fourths in 3 wholes. If 3 is $\frac{1}{4}$, the whole 12.

Explanation: Given that 3 ÷ $\frac{1}{4}$ which is 3 × 4 = 12. And there are 4 fourths in 1 whole and there are 12 fourths in 3 wholes.

c. 4 ÷ $\frac{1}{3}$ = _________ There are ____ thirds in 1 whole. There are ____ thirds in __ wholes. If 4 is $\frac{1}{3}$, what is the whole? _______

Answer: 4 ÷ $\frac{1}{3}$ = 12 There are 3 thirds in 1 whole. There are 12 thirds in 4 wholes. If 4 is $\frac{1}{3}$, the whole 12.

Explanation: Given that 4 ÷ $\frac{1}{3}$ which is 4 × 3 = 12. And there are 3 thirds in 1 whole and there are 12 thirds in 4 wholes.

d. 5 ÷ $\frac{1}{4}$ = _________ There are ____ fourths in 1 whole. There are ____ fourths in __ wholes. If 5 is $\frac{1}{4}$, what is the whole? _______

Answer: 5 ÷ $\frac{1}{4}$ = 20 There are 4 fourths in 1 whole. There are 20 fourths in 5 wholes. If 5 is $\frac{1}{4}$, the whole is 20.

Explanation: Given that 5 ÷ $\frac{1}{4}$ which is 5 × 4 = 20. And there are 4 fourths in 1 whole and there are 20 fourths in 5 wholes.

Question 2. Divide. Then, multiply to check.

a. 2 ÷ $\frac{1}{4}$

Answer: 2 ÷ $\frac{1}{4}$ = 8.

Explanation: Given that 2 ÷ $\frac{1}{4}$ which is 2 × 4 = 8. To check we will perform multiplication $\frac{1}{4}$ × 8 which is 2.

b. 6 ÷ $\frac{1}{2}$

Answer: 6 ÷ $\frac{1}{2}$ = 12.

Explanation: Given that 6 ÷ $\frac{1}{2}$ which is 6 × 2 = 12. To check we will perform multiplication $\frac{1}{2}$ × 12 which is 6.

c. 5 ÷ $\frac{1}{4}$

Answer: 5 ÷ $\frac{1}{4}$ = 20.

Explanation: Given that 5 ÷ $\frac{1}{4}$ which is 5 × 4 = 20. To check we will perform multiplication $\frac{1}{4}$ × 20 which is 5.

d. 5 ÷ $\frac{1}{8}$

Answer: 5 ÷ $\frac{1}{8}$ = 40.

Explanation: Given that 5 ÷ $\frac{1}{8}$ which is 5 × 8 = 40. To check we will perform multiplication $\frac{1}{8}$ × 40 which is 5.

e. 6 ÷ $\frac{1}{3}$

Answer: 6 ÷ $\frac{1}{3}$ = 18.

Explanation: Given that 6 ÷ $\frac{1}{3}$ which is 6 × 3 = 18. To check we will perform multiplication $\frac{1}{3}$ × 18 which is 6.

f. 3 ÷ $\frac{1}{6}$

Answer: 3 ÷ $\frac{1}{6}$ = 18.

Explanation: Given that 3 ÷ $\frac{1}{6}$ which is 3 × 6 = 18. To check we will perform multiplication $\frac{1}{6}$ × 18 which is 3.

g. 6 ÷ $\frac{1}{5}$

Answer: 6 ÷ $\frac{1}{5}$ = 30.

Explanation: Given that 6 ÷ $\frac{1}{5}$ which is 6 × 5 = 30. To check we will perform multiplication $\frac{1}{5}$ × 30 which is 6.

h. 6 ÷ $\frac{1}{10}$

Answer: 6 ÷ $\frac{1}{10}$ = 60.

Explanation: Given that 6 ÷ $\frac{1}{10}$ which is 6 × 10 = 60. To check we will perform multiplication $\frac{1}{10}$ × 60 which is 6.

Question 3. A principal orders 8 sub sandwiches for a teachers’ meeting. She cuts the subs into thirds and puts the mini-subs onto a tray. How many mini-subs are on the tray?

Answer: There will be 24 mini-subs are on the tray.

Explanation: Given there are 8 sub sandwiches for a teachers meeting and each sandwich cuts into third. So 1 ÷ $\frac{1}{3}$ which is 1 × 3 = 3 as there are 8 sub sandwiches, so there will be 8 × 3 = 24 mini-subs are on the tray.

Question 4. Some students prepare 3 different snacks. They make $\frac{1}{8}$ pound bags of nut mix, $\frac{1}{4}$ pound bags of cherries, and $\frac{1}{6}$ pound bags of dried fruit. If they buy 3 pounds of nut mix, 5 pounds of cherries, and 4 pounds of dried fruit, how many of each type of snack bag will they be able to make?

Answer: There are 24 nut mix, 20 cherry bags and 24 dried fruits.

Explanation: As some students prepare 3 different snacks and they make $\frac{1}{8}$ pound bags of nut mix and they will be able to make number of nut mix bag is 3 ÷ $\frac{1}{8}$ which is 3 × 8 = 24, and $\frac{1}{4}$ pound bags of cherries they will be able to make number of cherries bag is 5 ÷ $\frac{1}{4}$ which is 5 × 4 = 20 , and $\frac{1}{6}$ pound bags of dried fruit and they will be able to make number of bags of dried fruit is 4 ÷ $\frac{1}{6}$ which is 4 × 6 = 24. So there are 24 nut mix, 20 cherry bags and 24 dried fruits.